Total Surface Area and Volume of a Cylinder

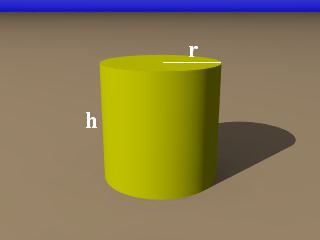
The total surface area is made up of a circular top and bottom, which are each the same size, and the side surface, which is actually a rectangle.

We must find the area of the top, or Area_top, which is the area of a circle with a radius of r.

This area is the same as the area of the bottom, so:

The area of the side is actually a rectangle. Imagine the cylinder to be a simple tin can. In your mind, cut off the top and the bottom and discard them. What is left is an open ended tube. Again in your mind, use some tin snips to cut along a straight line from top to bottom and uncurl and flatten out the tube. You would be looking at a rectangle that is as tall as the cylinder and as long as the circumference of its top (or bottom). Therefore, the area of the side is:

Now, since the circumference of the top is:

The area of the side is:

So, the total area of the cylinder is equal to the top area plus the bottom area plus the side area:

The volume of the cylinder, since it is a right solid, is the product of the top area times the height.

Example:

Let h = 5 cm, r = 2 cm

Area_top = (pi)(r²)

Area_top = (3.14)(2 cm)² = (3.14)4 cm² = 12.56 cm²

Area_bottom = Area_top = 12.56 cm²

Area_side = (h)(Circumference_top)

Area_side = (h)(2(pi)(r))

Area_side = 2(h)(pi)(r)

Area_side = 2(5 cm)(3.14)(2 cm)

Area_side = 62.8 cm²

Total Surface Area = Area_top = + Area_bottom + Area_side

Total Surface Area = 12.56 cm² + 12.56 cm² + 62.8 cm²

Total Surface Area = 87.92 cm²

Volume = (Area_top)(h)

Volume = (12.56 cm²)(5 cm)

Volume = 62.8 cm³